Polymer Physics

Kinetics of interior loop formation in semiflexible chains (JCP '06)

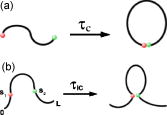
Loop formation between monomers in the interior of semiflexible chains describes elementary events in biomolecular folding and DNA bending. We calculate analytically the interior distance distribution function for semiflexible chains using a mean field approach. Using the potential of mean force derived from the distance distribution function we present a simple expression for the kinetics of interior looping by adopting Kramers theory. For the parameters, that are appropriate for DNA, the theoretical predictions in comparison with the case are in excellent agreement with explicit Brownian dynamics simulations of wormlike chain (WLC) model. The interior looping times (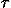_IC) can be greatly altered in the cases when the stiffness of the loop differs from that of the dangling ends. If the dangling end is stiffer than the loop then 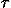_IC increases for the case of the WLC with uniform persistence length. In contrast, attachment of flexible dangling ends enhances rate of interior loop formation. The theory also shows that if the monomers are charged and interact via screened Coulomb potential then both the cyclization (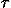_c) and interior looping (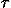_IC) times greatly increase at low ionic concentration. 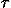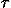Because both _c and _IC are determined essentially by the effective persistence length [l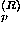] we computed l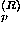 by varying the range of the repulsive interaction between the monomers. For short range interactions l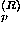 nearly coincides with the bare persistence length which is determined largely by the backbone chain connectivity. This finding rationalizes the efficacy of describing a number of experimental observations (response of biopolymers to force and cyclization kinetics) in biomolecules using WLC model with an effective persistence length.

Stretching homopolymers (Macromolecules '07)

Force-induced stretching of polymers is important in a variety of contexts. We have used theory and simulations to describe the response of homopolymers, with N monomers, to an external force (f ) in good and poor solvents. In good solvents and for sufficiently large N we show, in accord with scaling predictions, that the mean extension along the f axis <Z> ~ f for small f and <Z> ~ f ^2/3 (the Pincus regime) for intermediate values of f. The theoretical predictions for <Z> as a function of f are in excellent agreement with simulations for N = 100 and 1600. However, even with N = 1600, the expected Pincus regime is not observed due to the breakdown of the assumptions in the blob picture for finite N. We predict the Pincus scaling in a good solvent will be observed for N 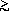10⁵. The force-dependent structure factors for a polymer in a poor solvent show that there is a hierarchy of structures, depending on the nature of the solvent. For a weakly hydrophobic polymer, various structures (ideal conformations, self-avoiding chains, globules, and rods) emerge on distinct length scales as f is varied. A strongly hydrophobic polymer remains globular as long as f is less than a critical value f_c. Above f_c, an abrupt first-order transition to a rodlike structure occurs. Our predictions can be tested using single molecule experiments.